A Sampling Theorem for Convex Shapes with Algebraic Boundaries

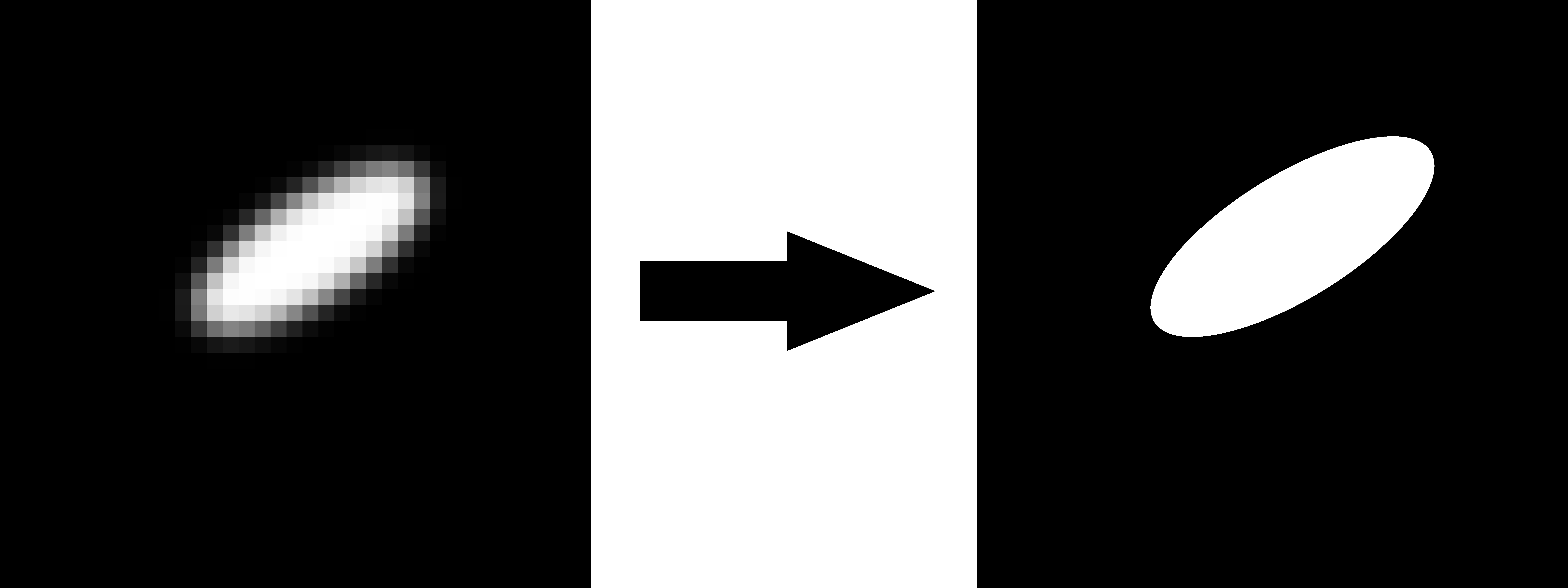
In this paper we present a sampling result about continuous-domain black and white images that form a convex shape. In particular, we will study shapes whose boundaries belong to the zero-level sets (roots) of bivariate polynomials. In [1] it was shown that generalized 2D moments of the image can lead to annihilation equations for the coefficients of the bivariate polynomial that determine the boundary of the shape. More precisely, when the bivariate polynomial is of degree n (which has n(n+3)/2 non-trivial coefficients), the results in [1] indicate that for invertibility of the linear annihilation equations, 2D moments of up to degree 3n−1 (overall equation moments) are required. In this paper, we improve this result for convex shapes by showing that 2D moments of up to degree 2n+1 (overall equation moments) are sufficient.

[Download paper here] [bibtex]